The normal distribution is a continuous probability distribution. The total area under the standard normal curve is always 1. The normal distribution looks like a bell-shaped curve.
In this article, we will discuss a step-by-step guide on finding the area under the standard normal curve to the left of the z score.
The area under the Standard Normal Curve to the left of z
Steps to find the area under the standard normal curve to the left of z
-
Step 1: Choose between a negative z-table or a positive z-table corresponding to the given z-score.
-
Step 2: Check the area value for the given z in the z-table.
-
Step 3: Look at the first two digits of the z-score on the left side column (y-axis) of the z-table and then the remaining number on the x-axis on the topmost row.
-
Step 4:The intersection of the two will be the required area.
-
Step 5: Multiply it by 100 to calculate the percentage of area.
Let’s understand find the area under the standard normal curve to the left of z using an example.
How do you find the area to the left of z = 1.55?
To find the area to the left of the given z follows the below steps:
-
Step 1: Choose a positive z-table as the given z-score (i.e 1.55) is positive.
-
Step 2: Check the area value for the given z in the z-table.
-
Step 3: Look at the first two digits (1.5) of the z-score on the left side column (y-axis) of the z-table and then the remaining number (0.05) on the x-axis on the topmost row.
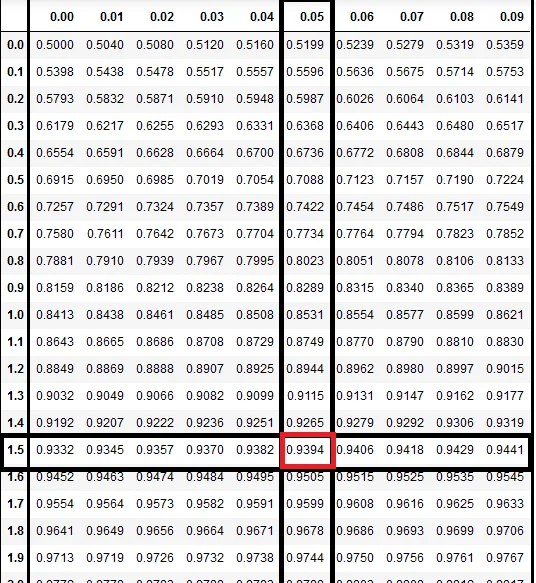
-
Step 4:The intersection of the two is 0.9394 (highlighted in red).
-
Step 5: Multiply it by 100 to calculate the percentage of area.
area to the left = 0.9394*100 = 93.94%
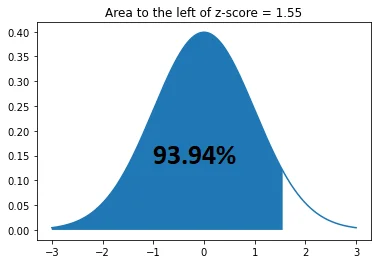
- Result: The area to the left of z is 93.94% of the normal standard curve.
Conclusion
I hope the above article to find the area under the standard normal curve to the left of z using step by step guide is helpful to you.
