In this blog post, we will discuss how to find the z-score for having an area 0.09 to the left of the standard normal curve.
To find the z-score for a given area, check if the area is left to the mean or right to the mean. Based on that, we will use either a negative z-table or a positive z-table.
How to Find the z score for having an area 0.09
Let’s find out the z-score for a given area of 0.09 using the below step-by-step guide.
-
Step 1: Symmetric property of the standard normal curve indicates 50% area lies to the left of the mean and 50% of the area lies to the right of the mean.
-
Step 2: Compare the given area with 0.50.
Given area = 0.09 < 0.50, indicates the area is left to the mean i.e. negative z-score.
-
Step 3: Choose a negative z-table as the required z-score is negative.
-
Step 4: Draw the normal distribution for the given area = 0.09
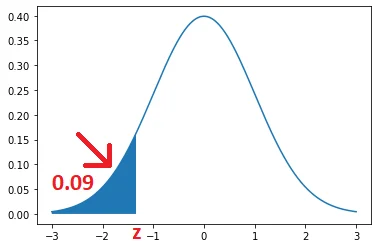
- Step 5: Trace the closest value to the given area=0.09 in the z-table.
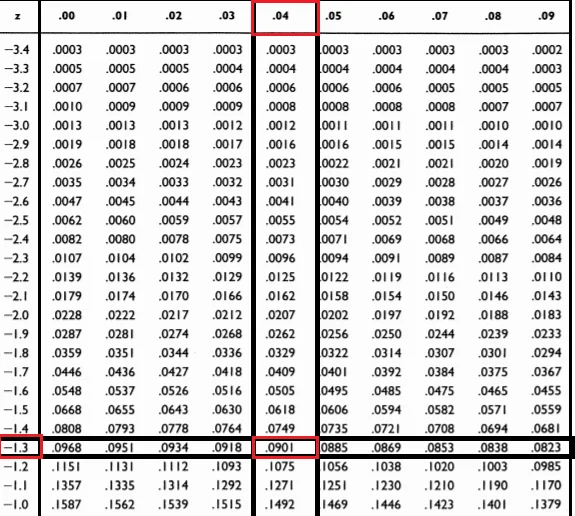
-
Step 6: Note the corresponding row and column values from z-table
-
Step 7: Combine the numbers to calculate the required z-score.
Z-score = row value + column value (as per z-table)
-1.3 + 0.04
Z-score = -1.34
Result: The Z-score for the area of 0.09 is -1.34.
Conclusion
I hope the above article to find the z-score for a given area 0.09 is helpful to you.
